DEMO_febio_0018_cube_poroelastic_ramp_hold
Below is a demonstration for:
- Building geometry for a cube with hexahedral elements
- Defining the boundary conditions
- Coding the febio structure
- Running the model
- Importing and visualizing the displacement and stress results
Contents
Keywords
- febio_spec version 4.0
- febio, FEBio
- uniaxial loading
- compression, tension, compressive, tensile
- displacement control, displacement boundary condition
- hexahedral elements, hex8
- cube, box, rectangular
- static, solid
- hyperelastic, Ogden
- viscoelastic
- poroelastic
- biphasic
- uncoupled, coupled
- ramp hold
- displacement logfile
- stress logfile
clear; close all; clc;
Plot settings
fontSize=20; faceAlpha1=0.8; markerSize=40; markerSize2=20; lineWidth=3;
Control parameters
% Path names defaultFolder = fileparts(fileparts(mfilename('fullpath'))); savePath=fullfile(defaultFolder,'data','temp'); % Defining file names febioFebFileNamePart='tempModel'; febioFebFileName=fullfile(savePath,[febioFebFileNamePart,'.feb']); %FEB file name febioLogFileName=[febioFebFileNamePart,'.txt']; %FEBio log file name febioLogFileName_disp=[febioFebFileNamePart,'_disp_out.txt']; %Log file name for exporting displacement febioLogFileName_stress=[febioFebFileNamePart,'_stress_out.txt']; %Log file name for exporting stress %Specifying dimensions and number of elements meshType='hex8'; %hex8 or tet4 unitSystem=2; %1=m, 2=mm switch unitSystem case 1 min_residual=1e-30; cubeSize=10e-3; pointSpacings=2e-3*ones(1,3); %Desired point spacing between nodes %Material parameter set E_youngs=1000; %Youngs modulus (Neo-Hookean) d=1000; %Density %Constant Isotropic Permeability parameters phi0=0.2; %Solid volume fraction in reference configuration permHydro=7.41e-11*100; %hydraulic permeability case 2 min_residual=1e-20; cubeSize=10; pointSpacings=2*ones(1,3); %Desired point spacing between nodes %Material parameter set E_youngs=1e-3; %Youngs modulus (Neo-Hookean) d=1e-9; %Density %Constant Isotropic Permeability parameters phi0=0.5; %Solid volume fraction in reference configuration permHydro=7.41e1*100; %hydraulic permeability end nu=0.4; %Material Poisson's ratio sampleWidth=cubeSize; %Width sampleThickness=cubeSize; %Thickness sampleHeight=cubeSize; %Height numElementsWidth=round(sampleWidth/pointSpacings(1)); %Number of elemens in dir 1 numElementsThickness=round(sampleThickness/pointSpacings(2)); %Number of elemens in dir 2 numElementsHeight=round(sampleHeight/pointSpacings(3)); %Number of elemens in dir 3 %Define applied displacement appliedStrain=0.4; %Linear strain (Only used to compute applied stretch) loadingOption='compression'; % or 'tension' switch loadingOption case 'compression' stretchLoad=1-appliedStrain; %The applied stretch for uniaxial loading case 'tension' stretchLoad=1+appliedStrain; %The applied stretch for uniaxial loading end displacementMagnitude=(stretchLoad*sampleHeight)-sampleHeight; %The displacement magnitude % FEA control settings analysisType='TRANSIENT';%'steady-state'; febioModule='biphasic'; t_load=0.1; %Time from start to max load t_step_ini1=t_load/50; %Initial desired step size numTimeSteps1=round(t_load/t_step_ini1); %Number of time steps desired t_step1=t_load/numTimeSteps1; %Step size dtmin1=t_step1/100; %Smallest allowed step size dtmax1=t_step1; %Largest allowed step size t_hold=1; t_step_ini2=t_hold/50; %Initial desired step size numTimeSteps2=round(t_hold/t_step_ini2); %Number of time steps desired t_step2=t_hold/numTimeSteps2; %Step size dtmin2=t_step2/100; %Smallest allowed step size dtmax2=t_step2; %Largest allowed step size max_refs=25; %Max reforms max_ups=0; %Set to zero to use full-Newton iterations opt_iter=6; %Optimum number of iterations max_retries=5; %Maximum number of retires runMode='external';% 'internal' or 'external'
Creating model geometry and mesh
A box is created with tri-linear hexahedral (hex8) elements using the hexMeshBox function. The function offers the boundary faces with seperate labels for the top, bottom, left, right, front, and back sides. As such these can be used to define boundary conditions on the exterior.
% Create a box with hexahedral elements cubeDimensions=[sampleWidth sampleThickness sampleHeight]; %Dimensions cubeElementNumbers=[numElementsWidth numElementsThickness numElementsHeight]; %Number of elements switch meshType case 'hex8' %hex8 structured [meshStruct]=hexMeshBox(cubeDimensions,cubeElementNumbers,2); case 'tet4' %tet4 unstructured [meshStruct]=tetMeshBox(cubeDimensions,mean(pointSpacings)); end %Access elements, nodes, and faces from the structure E=meshStruct.elements; %The elements V=meshStruct.nodes; %The nodes (vertices) Fb=meshStruct.facesBoundary; %The boundary faces Cb=meshStruct.boundaryMarker; %The "colors" or labels for the boundary faces elementMaterialIndices=ones(size(E,1),1); %Element material indices
Plotting model boundary surfaces and a cut view
hFig=cFigure; subplot(1,2,1); hold on; title('Model boundary surfaces and labels','FontSize',fontSize); gpatch(Fb,V,Cb,'k',faceAlpha1); colormap(gjet(6)); icolorbar; axisGeom(gca,fontSize); hs=subplot(1,2,2); hold on; title('Cut view of solid mesh','FontSize',fontSize); optionStruct.hFig=[hFig hs]; meshView(meshStruct,optionStruct); axisGeom(gca,fontSize); drawnow;

Defining the boundary conditions
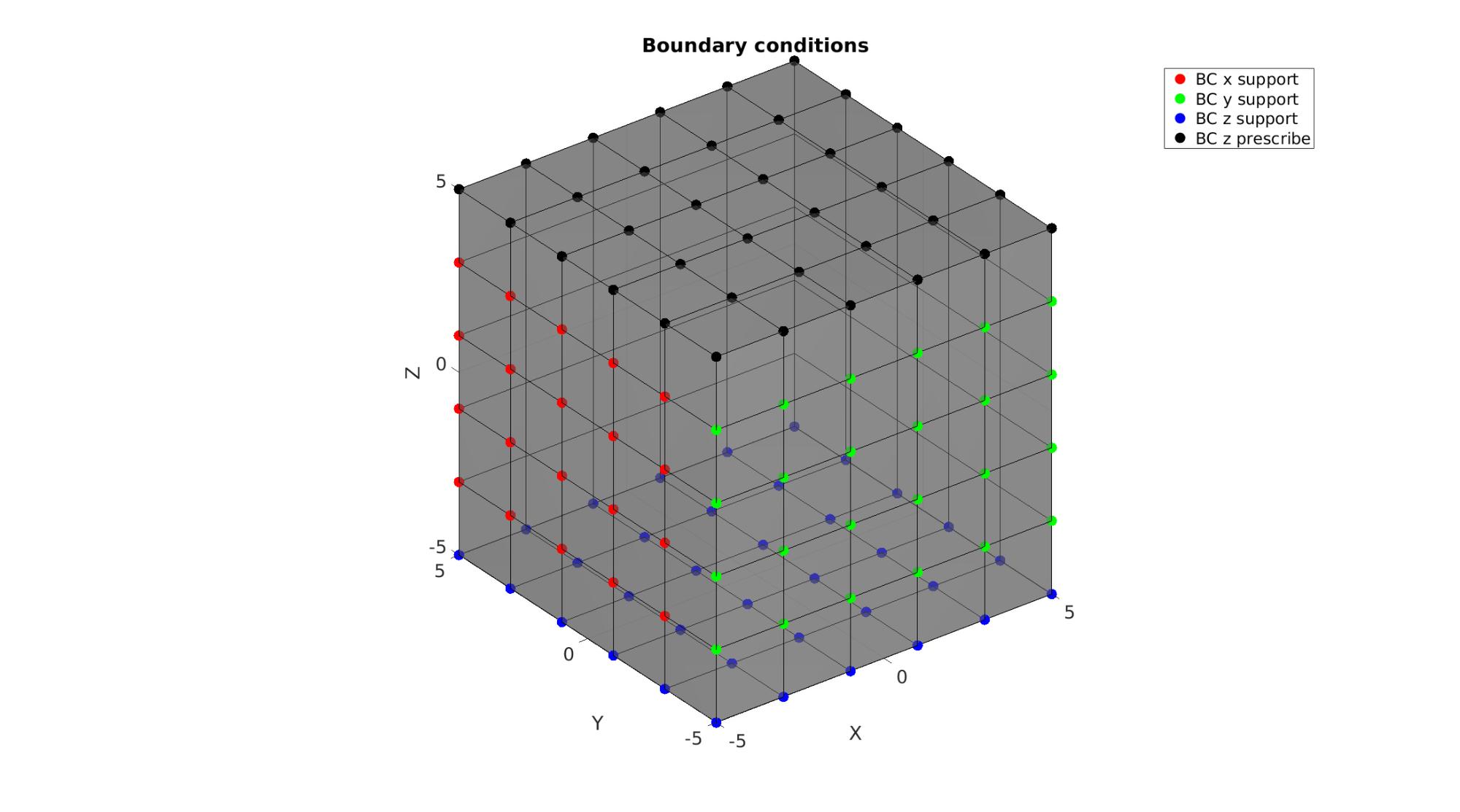
The visualization of the model boundary shows colors for each side of the cube. These labels can be used to define boundary conditions.
%Define supported node sets logicFace=Cb==1; %Logic for current face set Fr=Fb(logicFace,:); %The current face set bcSupportList_X=unique(Fr(:)); %Node set part of selected face logicFace=Cb==3; %Logic for current face set Fr=Fb(logicFace,:); %The current face set bcSupportList_Y=unique(Fr(:)); %Node set part of selected face logicFace=Cb==5; %Logic for current face set Fr=Fb(logicFace,:); %The current face set bcSupportList_Z=unique(Fr(:)); %Node set part of selected face %Prescribed displacement nodes logicPrescribe=Cb==6; %Logic for current face set Fr=Fb(logicPrescribe,:); %The current face set bcPrescribeList=unique(Fr(:)); %Node set part of selected face
Visualizing boundary conditions. Markers plotted on the semi-transparent model denote the nodes in the various boundary condition lists.
hf=cFigure; title('Boundary conditions','FontSize',fontSize); xlabel('X','FontSize',fontSize); ylabel('Y','FontSize',fontSize); zlabel('Z','FontSize',fontSize); hold on; gpatch(Fb,V,'kw','k',0.5); hl(1)=plotV(V(bcSupportList_X,:),'r.','MarkerSize',markerSize); hl(2)=plotV(V(bcSupportList_Y,:),'g.','MarkerSize',markerSize); hl(3)=plotV(V(bcSupportList_Z,:),'b.','MarkerSize',markerSize); hl(4)=plotV(V(bcPrescribeList,:),'k.','MarkerSize',markerSize); legend(hl,{'BC x support','BC y support','BC z support','BC z prescribe'}); axisGeom(gca,fontSize); camlight headlight; drawnow;
Defining the FEBio input structure
See also febioStructTemplate and febioStruct2xml and the FEBio user manual.
%Get a template with default settings [febio_spec]=febioStructTemplate; %febio_spec version febio_spec.ATTR.version='4.0'; %Module section febio_spec.Module.ATTR.type=febioModule; %Control sections for each step febio_spec.Step.step{1}.Control=febio_spec.Control; %Copy from template febio_spec.Step.step{1}.ATTR.id=1; febio_spec.Step.step{1}.ATTR.name='Step01'; febio_spec.Step.step{1}.Control.analysis=analysisType; febio_spec.Step.step{1}.Control.time_steps=numTimeSteps1; febio_spec.Step.step{1}.Control.step_size=t_step1; febio_spec.Step.step{1}.Control.solver.ATTR.type=febioModule; febio_spec.Step.step{1}.Control.solver.max_refs=max_refs; febio_spec.Step.step{1}.Control.solver.symmetric_stiffness=0; %Recommended for biphasic analysis febio_spec.Step.step{1}.Control.solver.min_residual=min_residual; febio_spec.Step.step{1}.Control.time_stepper.dtmin=dtmin1; febio_spec.Step.step{1}.Control.time_stepper.dtmax=dtmax1; febio_spec.Step.step{1}.Control.time_stepper.max_retries=max_retries; febio_spec.Step.step{1}.Control.time_stepper.opt_iter=opt_iter; febio_spec.Step.step{2}.Control=febio_spec.Control; %Copy from template febio_spec.Step.step{2}.ATTR.id=2; febio_spec.Step.step{2}.ATTR.name='Step02'; febio_spec.Step.step{2}.Control.analysis=analysisType; febio_spec.Step.step{2}.Control.time_steps=numTimeSteps2; febio_spec.Step.step{2}.Control.step_size=t_step2; febio_spec.Step.step{2}.Control.solver.ATTR.type=febioModule; febio_spec.Step.step{2}.Control.solver.max_refs=max_refs; febio_spec.Step.step{2}.Control.solver.symmetric_stiffness=0; %Recommended for biphasic analysis febio_spec.Step.step{2}.Control.solver.min_residual=min_residual; febio_spec.Step.step{2}.Control.time_stepper.dtmin=dtmin2; febio_spec.Step.step{2}.Control.time_stepper.dtmax=dtmax2; febio_spec.Step.step{2}.Control.time_stepper.max_retries=max_retries; febio_spec.Step.step{2}.Control.time_stepper.opt_iter=opt_iter; %Remove control field (part of template) since step specific control sections are used febio_spec=rmfield(febio_spec,'Control'); %Material section %Viscous part materialName1='Material1'; febio_spec.Material.material{1}.ATTR.name=materialName1; febio_spec.Material.material{1}.ATTR.type='biphasic'; febio_spec.Material.material{1}.ATTR.id=1; febio_spec.Material.material{1}.phi0=phi0; febio_spec.Material.material{1}.fluid_density=d; febio_spec.Material.material{1}.tau=0; %Solid part febio_spec.Material.material{1}.solid.ATTR.type='neo-Hookean'; febio_spec.Material.material{1}.solid.density=1; febio_spec.Material.material{1}.solid.E=E_youngs; febio_spec.Material.material{1}.solid.v=nu; %Permeability part febio_spec.Material.material{1}.permeability.ATTR.type='perm-const-iso'; febio_spec.Material.material{1}.permeability.perm=permHydro; % Mesh section % -> Nodes febio_spec.Mesh.Nodes{1}.ATTR.name='Object1'; %The node set name febio_spec.Mesh.Nodes{1}.node.ATTR.id=(1:size(V,1))'; %The node id's febio_spec.Mesh.Nodes{1}.node.VAL=V; %The nodel coordinates % -> Elements partName1='Part1'; febio_spec.Mesh.Elements{1}.ATTR.name=partName1; %Name of this part febio_spec.Mesh.Elements{1}.ATTR.type=meshType; %Element type febio_spec.Mesh.Elements{1}.elem.ATTR.id=(1:1:size(E,1))'; %Element id's febio_spec.Mesh.Elements{1}.elem.VAL=E; %The element matrix % -> NodeSets nodeSetName1='bcSupportList_X'; nodeSetName2='bcSupportList_Y'; nodeSetName3='bcSupportList_Z'; nodeSetName4='bcPrescribeList'; febio_spec.Mesh.NodeSet{1}.ATTR.name=nodeSetName1; febio_spec.Mesh.NodeSet{1}.VAL=mrow(bcSupportList_X); febio_spec.Mesh.NodeSet{2}.ATTR.name=nodeSetName2; febio_spec.Mesh.NodeSet{2}.VAL=mrow(bcSupportList_Y); febio_spec.Mesh.NodeSet{3}.ATTR.name=nodeSetName3; febio_spec.Mesh.NodeSet{3}.VAL=mrow(bcSupportList_Z); febio_spec.Mesh.NodeSet{4}.ATTR.name=nodeSetName4; febio_spec.Mesh.NodeSet{4}.VAL=mrow(bcPrescribeList); %MeshDomains section febio_spec.MeshDomains.SolidDomain.ATTR.name=partName1; febio_spec.MeshDomains.SolidDomain.ATTR.mat=materialName1; %Boundary condition section % -> Fix boundary conditions febio_spec.Boundary.bc{1}.ATTR.name='FixedDisplacement01'; febio_spec.Boundary.bc{1}.ATTR.type='zero displacement'; febio_spec.Boundary.bc{1}.ATTR.node_set=nodeSetName1; febio_spec.Boundary.bc{1}.x_dof=1; febio_spec.Boundary.bc{1}.y_dof=0; febio_spec.Boundary.bc{1}.z_dof=0; febio_spec.Boundary.bc{2}.ATTR.name='FixedDisplacement02'; febio_spec.Boundary.bc{2}.ATTR.type='zero displacement'; febio_spec.Boundary.bc{2}.ATTR.node_set=nodeSetName2; febio_spec.Boundary.bc{2}.x_dof=0; febio_spec.Boundary.bc{2}.y_dof=1; febio_spec.Boundary.bc{2}.z_dof=0; febio_spec.Boundary.bc{3}.ATTR.name='FixedDisplacement03'; febio_spec.Boundary.bc{3}.ATTR.type='zero displacement'; febio_spec.Boundary.bc{3}.ATTR.node_set=nodeSetName3; febio_spec.Boundary.bc{3}.x_dof=0; febio_spec.Boundary.bc{3}.y_dof=0; febio_spec.Boundary.bc{3}.z_dof=1; febio_spec.Boundary.bc{4}.ATTR.name='FixedFluidPressure01'; febio_spec.Boundary.bc{4}.ATTR.type='zero fluid pressure'; febio_spec.Boundary.bc{4}.ATTR.node_set=nodeSetName4; febio_spec.Boundary.bc{5}.ATTR.name='bcPrescribeListZ'; febio_spec.Boundary.bc{5}.ATTR.type='prescribed displacement'; febio_spec.Boundary.bc{5}.ATTR.node_set=nodeSetName4; febio_spec.Boundary.bc{5}.dof='z'; febio_spec.Boundary.bc{5}.value.ATTR.lc=1; febio_spec.Boundary.bc{5}.value.VAL=displacementMagnitude; febio_spec.Boundary.bc{5}.relative=0; %LoadData section % -> load_controller febio_spec.LoadData.load_controller{1}.ATTR.name='LC1'; febio_spec.LoadData.load_controller{1}.ATTR.id=1; febio_spec.LoadData.load_controller{1}.ATTR.type='loadcurve'; febio_spec.LoadData.load_controller{1}.interpolate='LINEAR'; febio_spec.LoadData.load_controller{1}.extend='CONSTANT'; febio_spec.LoadData.load_controller{1}.points.pt.VAL=[0 0;t_load 1;(t_load+t_hold) 1]; %Output section % -> log file febio_spec.Output.logfile.ATTR.file=febioLogFileName; febio_spec.Output.logfile.node_data{1}.ATTR.file=febioLogFileName_disp; febio_spec.Output.logfile.node_data{1}.ATTR.data='ux;uy;uz'; febio_spec.Output.logfile.node_data{1}.ATTR.delim=','; febio_spec.Output.logfile.element_data{1}.ATTR.file=febioLogFileName_stress; febio_spec.Output.logfile.element_data{1}.ATTR.data='sz'; febio_spec.Output.logfile.element_data{1}.ATTR.delim=','; febio_spec.Output.plotfile.compression=0;
Quick viewing of the FEBio input file structure
The febView function can be used to view the xml structure in a MATLAB figure window.
febView(febio_spec); %Viewing the febio file
Exporting the FEBio input file
Exporting the febio_spec structure to an FEBio input file is done using the febioStruct2xml function.
febioStruct2xml(febio_spec,febioFebFileName); %Exporting to file and domNode
Running the FEBio analysis
To run the analysis defined by the created FEBio input file the runMonitorFEBio function is used. The input for this function is a structure defining job settings e.g. the FEBio input file name. The optional output runFlag informs the user if the analysis was run succesfully.
febioAnalysis.run_filename=febioFebFileName; %The input file name febioAnalysis.run_logname=febioLogFileName; %The name for the log file febioAnalysis.disp_on=1; %Display information on the command window febioAnalysis.runMode=runMode; [runFlag]=runMonitorFEBio(febioAnalysis);%START FEBio NOW!!!!!!!!
%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
--------> RUNNING/MONITORING FEBIO JOB <-------- 20-Apr-2023 10:42:34
FEBio path: /home/kevin/FEBioStudio2/bin/febio4
# Attempt removal of existing log files 20-Apr-2023 10:42:34
* Removal succesful 20-Apr-2023 10:42:34
# Attempt removal of existing .xplt files 20-Apr-2023 10:42:35
* Removal succesful 20-Apr-2023 10:42:35
# Starting FEBio... 20-Apr-2023 10:42:35
Max. total analysis time is: Inf s
* Waiting for log file creation 20-Apr-2023 10:42:35
Max. wait time: 30 s
* Log file found. 20-Apr-2023 10:42:35
# Parsing log file... 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.002 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.004 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.006 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.008 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.01 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.012 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:35
number of reformations : 3 20-Apr-2023 10:42:35
------- converged at time : 0.014 20-Apr-2023 10:42:35
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.016 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.018 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.02 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.022 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.024 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.026 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.028 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.03 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.032 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.034 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.036 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.038 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.04 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.042 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.044 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.046 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.048 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:36
number of reformations : 3 20-Apr-2023 10:42:36
------- converged at time : 0.05 20-Apr-2023 10:42:36
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.052 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.054 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.056 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.058 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.06 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.062 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.064 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.066 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.068 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.07 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.072 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.074 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.076 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.078 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.08 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.082 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.084 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.086 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:37
number of reformations : 3 20-Apr-2023 10:42:37
------- converged at time : 0.088 20-Apr-2023 10:42:37
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.09 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.092 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.094 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.096 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.098 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.1 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.12 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.14 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.16 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.18 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.2 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.22 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.24 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.26 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.28 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.3 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.32 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.34 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.36 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.38 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.4 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:38
number of reformations : 3 20-Apr-2023 10:42:38
------- converged at time : 0.42 20-Apr-2023 10:42:38
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.44 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.46 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.48 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.5 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.52 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.54 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.56 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.58 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.6 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.62 20-Apr-2023 10:42:39
number of iterations : 3 20-Apr-2023 10:42:39
number of reformations : 3 20-Apr-2023 10:42:39
------- converged at time : 0.64 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.66 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.68 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.7 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.72 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.74 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.76 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.78 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.8 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.82 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.84 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.86 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.88 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.9 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.92 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.94 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.96 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 0.98 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
number of reformations : 2 20-Apr-2023 10:42:39
------- converged at time : 1 20-Apr-2023 10:42:39
number of iterations : 2 20-Apr-2023 10:42:39
------- converged at time : 1.02 20-Apr-2023 10:42:40
number of iterations : 2 20-Apr-2023 10:42:40
number of reformations : 2 20-Apr-2023 10:42:40
------- converged at time : 1.04 20-Apr-2023 10:42:40
number of iterations : 2 20-Apr-2023 10:42:40
number of reformations : 2 20-Apr-2023 10:42:40
------- converged at time : 1.06 20-Apr-2023 10:42:40
number of iterations : 2 20-Apr-2023 10:42:40
number of reformations : 2 20-Apr-2023 10:42:40
------- converged at time : 1.08 20-Apr-2023 10:42:40
number of iterations : 2 20-Apr-2023 10:42:40
number of reformations : 2 20-Apr-2023 10:42:40
------- converged at time : 1.1 20-Apr-2023 10:42:40
Elapsed time : 0:00:05 20-Apr-2023 10:42:40
N O R M A L T E R M I N A T I O N
# Done 20-Apr-2023 10:42:40
%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%%
Import FEBio results
if runFlag==1 %i.e. a succesful run
Importing nodal displacements from a log file
dataStruct=importFEBio_logfile(fullfile(savePath,febioLogFileName_disp),0,1);
%Access data
N_disp_mat=dataStruct.data; %Displacement
timeVec=dataStruct.time; %Time
%Create deformed coordinate set
V_DEF=N_disp_mat+repmat(V,[1 1 size(N_disp_mat,3)]);
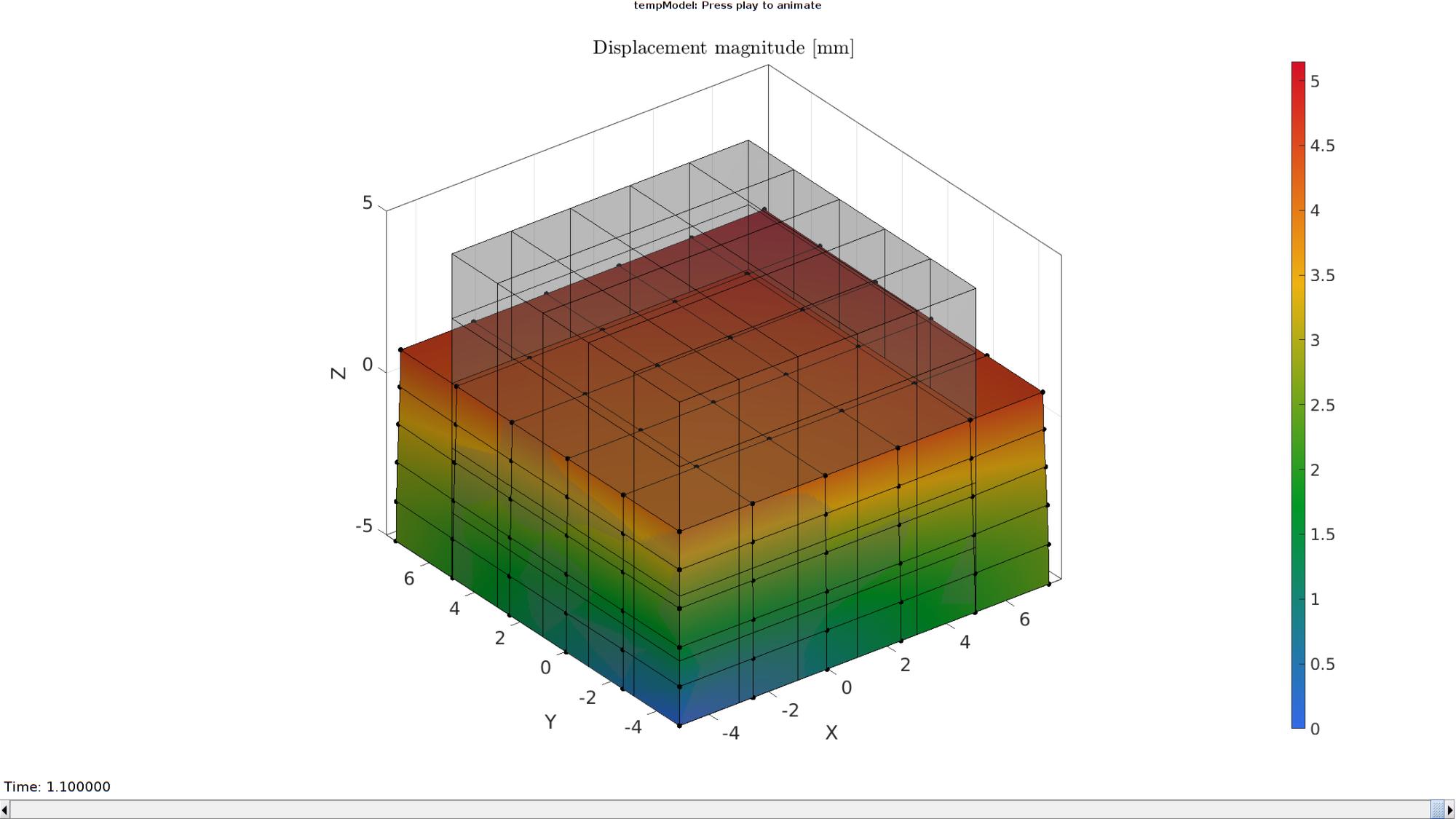
Plotting the simulated results using anim8 to visualize and animate deformations
DN_magnitude=sqrt(sum(N_disp_mat(:,:,end).^2,2)); %Current displacement magnitude % Create basic view and store graphics handle to initiate animation hf=cFigure; %Open figure gtitle([febioFebFileNamePart,': Press play to animate']); title('Displacement magnitude [mm]','Interpreter','Latex') hp=gpatch(Fb,V_DEF(:,:,end),DN_magnitude,'k',1); %Add graphics object to animate hp.Marker='.'; hp.MarkerSize=markerSize2; hp.FaceColor='interp'; gpatch(Fb,V,0.5*ones(1,3),'k',0.25); %A static graphics object axisGeom(gca,fontSize); colormap(gjet(250)); colorbar; caxis([0 max(DN_magnitude)]); axis(axisLim(V_DEF)); %Set axis limits statically camlight headlight; % Set up animation features animStruct.Time=timeVec; %The time vector for qt=1:1:size(N_disp_mat,3) %Loop over time increments DN_magnitude=sqrt(sum(N_disp_mat(:,:,qt).^2,2)); %Current displacement magnitude %Set entries in animation structure animStruct.Handles{qt}=[hp hp]; %Handles of objects to animate animStruct.Props{qt}={'Vertices','CData'}; %Properties of objects to animate animStruct.Set{qt}={V_DEF(:,:,qt),DN_magnitude}; %Property values for to set in order to animate end anim8(hf,animStruct); %Initiate animation feature drawnow;
Importing element stress from a log file
dataStruct=importFEBio_logfile(fullfile(savePath,febioLogFileName_stress),0,1);
%Access data
E_stress_mat=dataStruct.data;
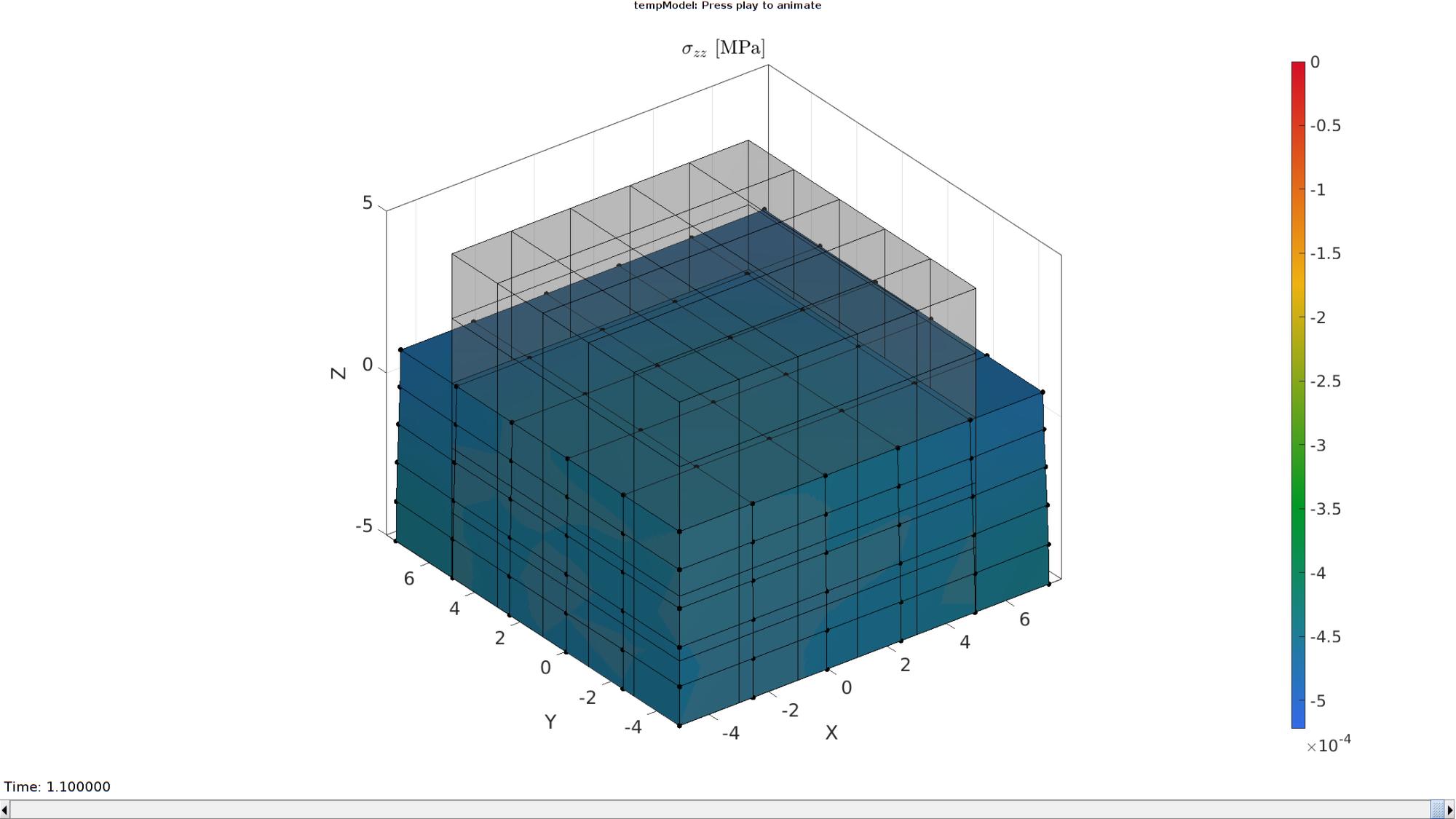
Plotting the simulated results using anim8 to visualize and animate deformations
[CV]=faceToVertexMeasure(E,V,E_stress_mat(:,:,end));
% Create basic view and store graphics handle to initiate animation
hf=cFigure; %Open figure
gtitle([febioFebFileNamePart,': Press play to animate']);
title('$\sigma_{zz}$ [MPa]','Interpreter','Latex')
hp=gpatch(Fb,V_DEF(:,:,end),CV,'k',1); %Add graphics object to animate
hp.Marker='.';
hp.MarkerSize=markerSize2;
hp.FaceColor='interp';
gpatch(Fb,V,0.5*ones(1,3),'k',0.25); %A static graphics object
axisGeom(gca,fontSize);
colormap(gjet(250)); colorbar;
caxis([min(E_stress_mat(:)) max(E_stress_mat(:))]);
axis(axisLim(V_DEF)); %Set axis limits statically
camlight headlight;
% Set up animation features
animStruct.Time=timeVec; %The time vector
for qt=1:1:size(N_disp_mat,3) %Loop over time increments
[CV]=faceToVertexMeasure(E,V,E_stress_mat(:,:,qt));
%Set entries in animation structure
animStruct.Handles{qt}=[hp hp]; %Handles of objects to animate
animStruct.Props{qt}={'Vertices','CData'}; %Properties of objects to animate
animStruct.Set{qt}={V_DEF(:,:,qt),CV}; %Property values for to set in order to animate
end
anim8(hf,animStruct); %Initiate animation feature
drawnow;
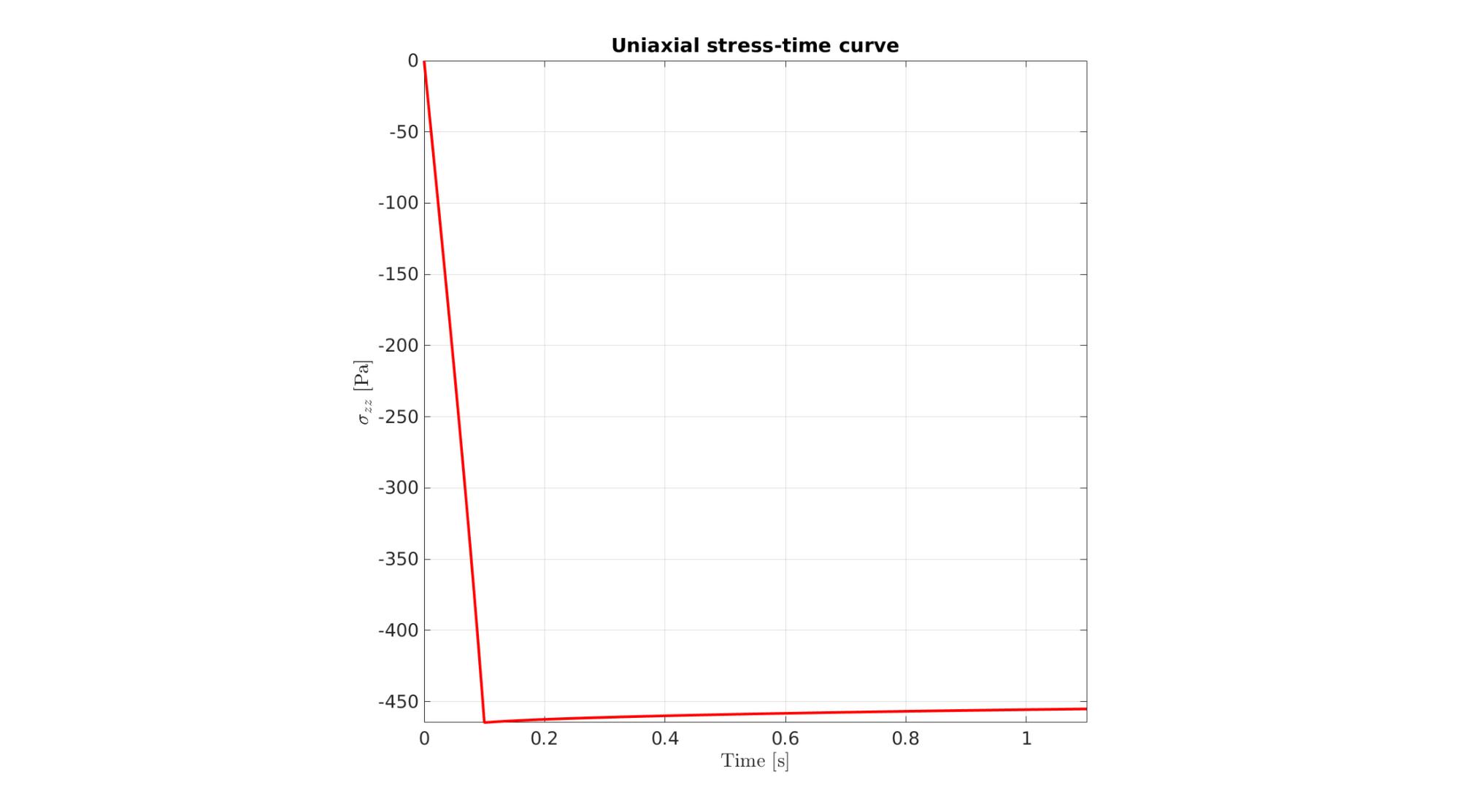
Calculate metrics to visualize time-stress curve
stress_cauchy_sim=mean(squeeze(E_stress_mat),1)';
Visualize stress-stretch curve
cFigure; hold on; title('Uniaxial stress-time curve','FontSize',fontSize); xlabel('Time [s]','FontSize',fontSize,'Interpreter','Latex'); ylabel('$\sigma_{zz}$ [Pa]','FontSize',fontSize,'Interpreter','Latex'); if unitSystem==1 plot(timeVec(:),stress_cauchy_sim(:),'r-','lineWidth',lineWidth); else %MPa -> Pa plot(timeVec(:),stress_cauchy_sim(:)*1e6,'r-','lineWidth',lineWidth); end view(2); axis tight; grid on; axis square; box on; set(gca,'FontSize',fontSize); drawnow;
end

GIBBON www.gibboncode.org
Kevin Mattheus Moerman, [email protected]
GIBBON footer text
License: https://github.com/gibbonCode/GIBBON/blob/master/LICENSE
GIBBON: The Geometry and Image-based Bioengineering add-On. A toolbox for image segmentation, image-based modeling, meshing, and finite element analysis.
Copyright (C) 2006-2022 Kevin Mattheus Moerman and the GIBBON contributors
This program is free software: you can redistribute it and/or modify it under the terms of the GNU General Public License as published by the Free Software Foundation, either version 3 of the License, or (at your option) any later version.
This program is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE. See the GNU General Public License for more details.
You should have received a copy of the GNU General Public License along with this program. If not, see http://www.gnu.org/licenses/.