DEMO_geodesic_remeshing

Below is a demonstration for:

Contents

clear; close all; clc;

%Plot settings
cMapDist=flipud(igviridis(250));
[cMapIndices,scrambleIndices]=scramble(viridis(250),1); %Colormap

faceAlpha1=1;
faceAlpha2=0.65;
fontSize=25;
markerSize=50;
lineWidth=4;
scatterSize=65;

Example 1: Resampling a triangulated surface model

Example: Using meshDistMarch for geodesic point sampling

Get example triangulated mesh data

[F,V]=graphicsModels(7);

Visualize input mesh

cFigure; hold on;
title('Input mesh')
gpatch(F,V,'gw');
axisGeom(gca,fontSize);
camlight headlight;
drawnow;

Compute distances on mesh

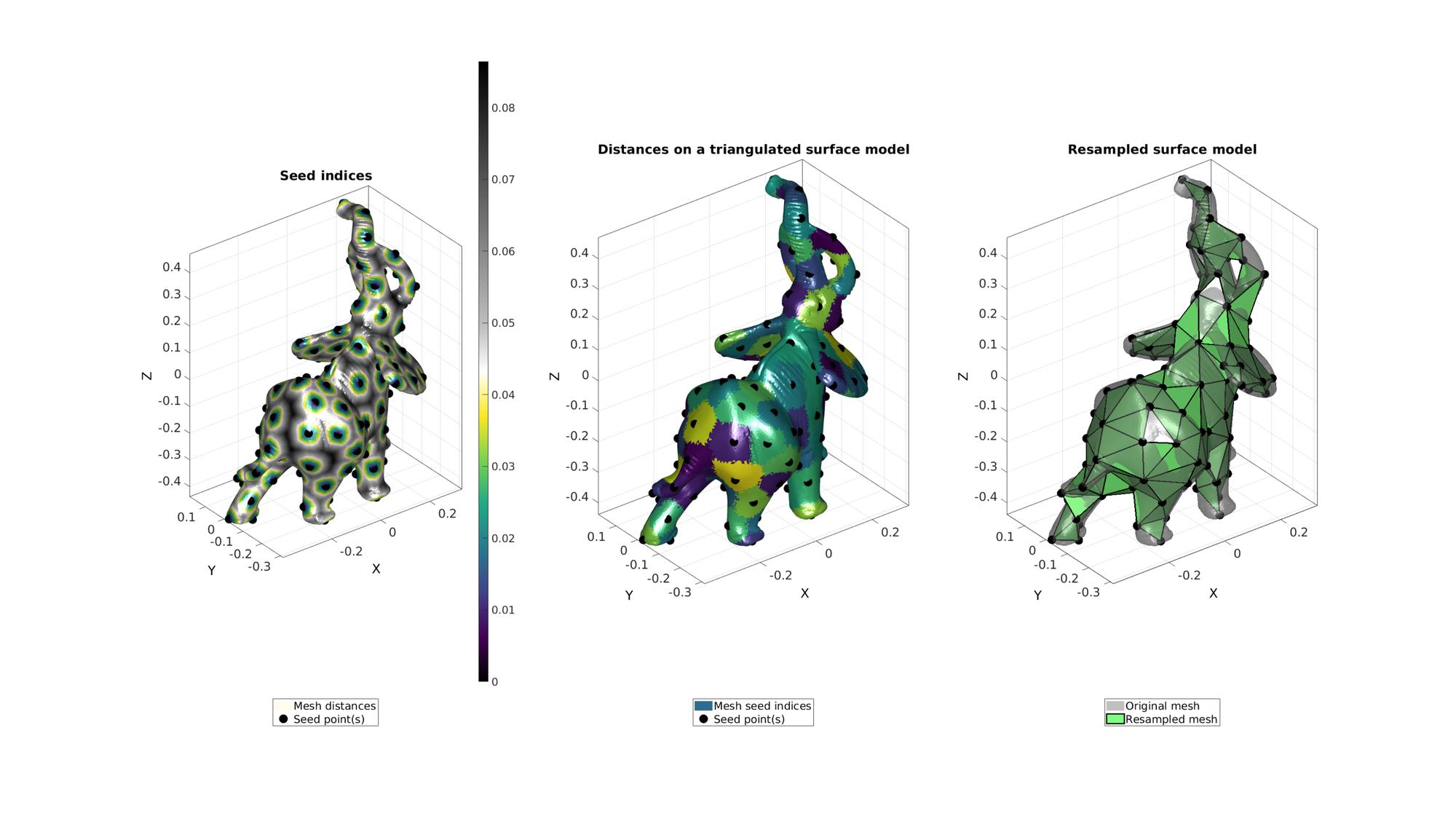
numSeeds=120;

%Option set
[~,indStart]=min(V(:,1)); %Index of the start point
optionStruct.toleranceLevel=0; %Tolerance for convergence
optionStruct.numSeeds=numSeeds; %Number of seeds
optionStruct.waitBarOn=1; %Turn on/off waitbar

%Compute distances on mesh description
[Fn,Vn,seedIndex,indSeeds,d]=remeshTriSurfDistMap(F,V,numSeeds,indStart,optionStruct); %distance based marching
[~,~,ind2]=unique(seedIndex);

Visualization

cFigure;
subplot(1,3,1); hold on;
title('Seed indices','fontSize',fontSize);
hp(1)=gpatch(F,V,d,'none',1); hp(1).FaceColor='Interp';
hp(2)=plotV(V(indSeeds,:),'k.','MarkerSize',markerSize);
legend(hp,{'Mesh distances','Seed point(s)'},'Location','SouthOutSide');
axisGeom;
camlight headlight;
colormap(gca,cMapDist); colorbar;

subplot(1,3,2); hold on;
title('Distances on a triangulated surface model','fontSize',fontSize);
hp(1)=gpatch(F,V,ind2,'none',1);
hp(2)=plotV(V(indSeeds,:),'k.','MarkerSize',markerSize);
legend(hp,{'Mesh seed indices','Seed point(s)'},'Location','SouthOutSide');
axisGeom;
camlight headlight;
colormap(gca,cMapIndices);

subplot(1,3,3); hold on;
title('Resampled surface model','fontSize',fontSize);
plotV(V(indSeeds,:),'k.','MarkerSize',50);
hp(1)=gpatch(F,V,'kw','none',0.5);
hp(2)=gpatch(Fn,Vn,'gw','k',1,2);
legend(hp,{'Original mesh','Resampled mesh'},'Location','SouthOutSide');

axisGeom;
camlight headlight;
drawnow;

Example 2: Boundary preserving resampling

Control parameters

pointSpacing=1; %Desired point spacing
nRefineOriginal=1; %Set n>0 to refine the input mesh through sub-triangulation before resampling
nRefineOutput=0; %Number of output refinement steps

Creating example surface data

%Boundary 1
ns=150;
t=linspace(0,2*pi,ns);
t=t(1:end-1);
r=6+2.*sin(5*t);
[x,y] = pol2cart(t,r);
z=1/10*x.^2;
V1=[x(:) y(:) z(:)];

%Create Euler angles to set directions
E=[0.25*pi -0.25*pi 0];
[R,~]=euler2DCM(E); %The true directions for X, Y and Z axis

V1=(R*V1')'; %Rotate polygon

regionCell={V1}; %A region between V1 and V2 (V2 forms a hole inside V1)

% Meshing the region (See also |regionTriMesh2D|)
[F,V]=regionTriMesh3D(regionCell,0.15,1,'natural');

Refine input mesh before resampling

Refining the input mesh is required if the intended mesh density exceeds that of the desired output mesh density. For each iteration the triangle edges are split in half while the triangle faces are split into 4. The mesh becomes very dense, very quickly so do not over do this. Subtri works through triangle splitting and is linear in the sense that it leaves input points unaltered but adds intermediate points on all input edges.

if nRefineOriginal>0
    for q=1:1:nRefineOriginal
        [F,V]=subtri(F,V,1); %Refine input mesh through sub-triangulation
    end
end

Estimate number of points required given point spacing

numPointsInput=size(V,1); %Number of points in the original data
[A]=patch_area(F,V); %Areas of current faces
totalArea=sum(A(:)); %Total area
l=sqrt(totalArea); %Width or length of square with same size
np=round((l./pointSpacing).^2); %Point spacing for mesh in virtual square

Get indices of boundary points and get boundary curve

%Boundary edges
Eb=patchBoundary(F,V);

%Reorder edge list to obtain indices describing continuous curve (assuming
%a single boundary, need to group first and do reordering on each group if
%multiple boundaries exist)
[indList]=edgeListToCurve(Eb);
indList=indList(1:end-1);

Get indices of "must points" close to evenly spaced on boundary curve

D=pathLength(V(indList,:)); %The cummulative curve length
boundaryLength=max(D); %The total curve length

nb=round(boundaryLength./pointSpacing); %Number of points to keep on boundary
[Vb] = evenlySampleCurve(V(indList,:),nb,'pchip',1);

[~,minIND]=minDist(Vb,V(indList,:));
indListSelect=indList(minIND); %List of points to keep

Visualize boundary points to keep on input mesh

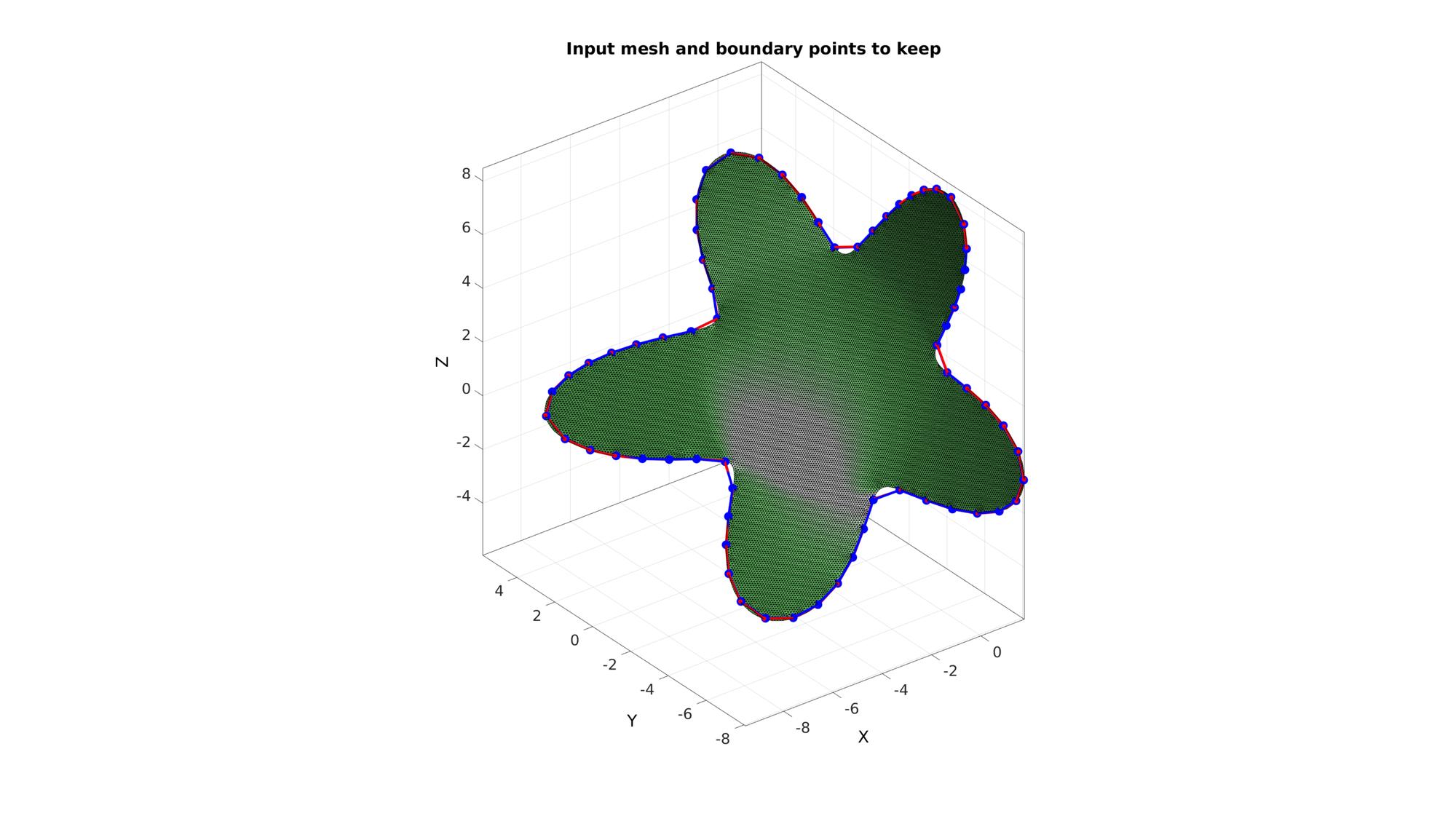
cFigure; hold on;
title('Input mesh and boundary points to keep')
gpatch(F,V,'gw');

plotV(V(indListSelect,:),'r.-','MarkerSize',25,'LineWidth',lineWidth);
plotV(Vb,'b.-','MarkerSize',markerSize,'LineWidth',lineWidth);

axisGeom(gca,fontSize);
camlight headlight;
drawnow;

Resample input surface geodesically

Geodesic re-sampling works by taking a point on the surface and calculating the distance to all other points by marching/propagating across the mesh. The point on the mesh furthest away from the input point (or points) is then added to the list. Then new distances are computed and the furthest point is again added to this list. Therefore using this iterative process equally spaced point sets can be obtained. The mesh works by sampling the input mesh to a coarser homogeneous mesh. If the input mesh is too coarse refine it first using subTri (subTri does not alter input geometry). This method does not alter the geometry but simply samples a subset of the input points. So the output point set (or seeds) are all points part of the original input geometry. The region closest to one of the seed points can be viewed as a Voronoi cell. The dual of the Voronoi tesselation is a Delaunay triangulation which gives the output mesh connectivity. The distance marching can be very slow. One tip is to do a coarse resampling and then to sub-triangulate the output.

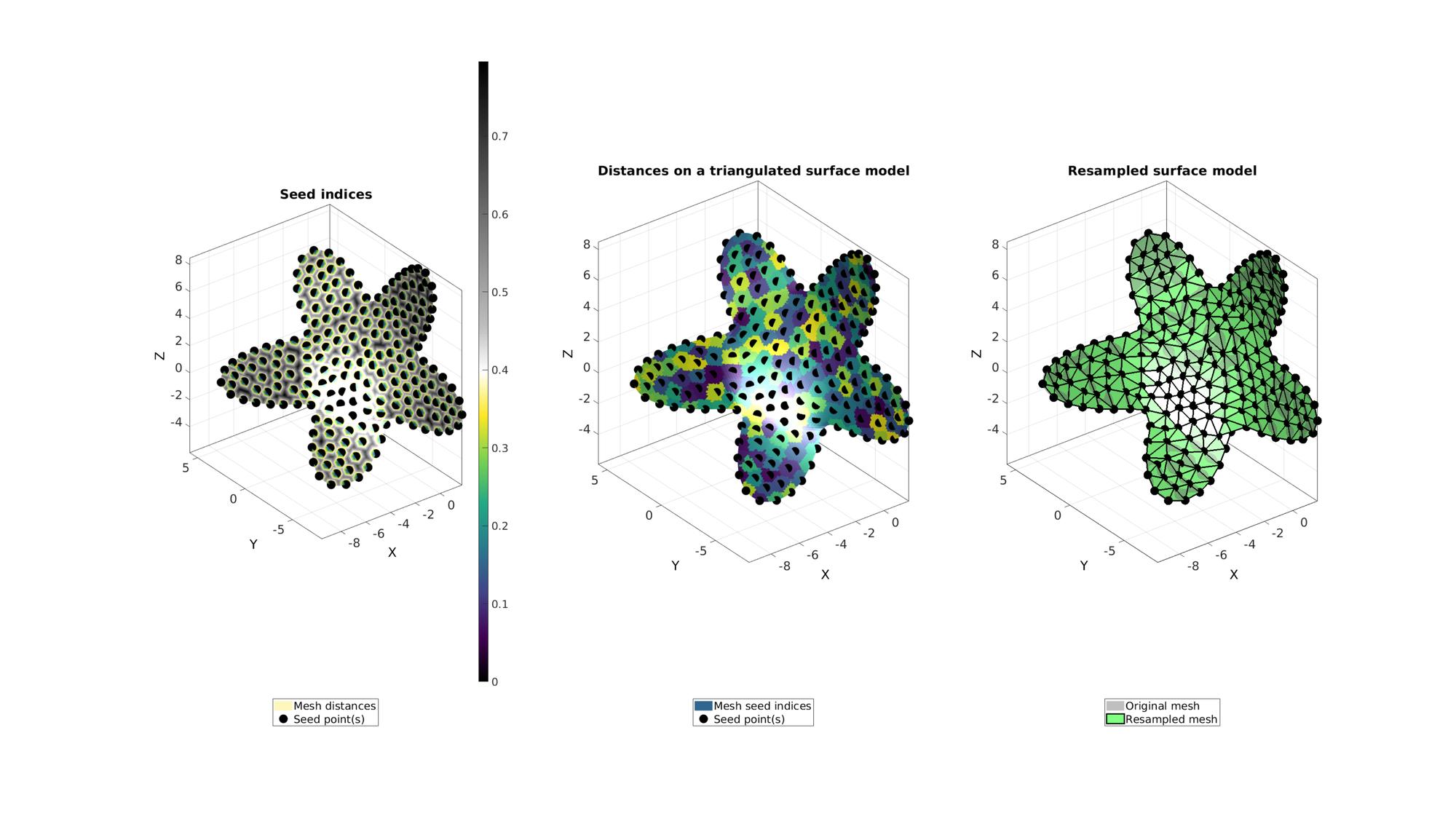
%Use distance marching method
clear optionStruct
optionStruct.toleranceLevel=0; %Tolerance for convergence
optionStruct.waitBarOn=1; %Turn on/off waitbar
[Fn,Vn,seedIndex,indSeeds,d]=remeshTriSurfDistMap(F,V,numel(indListSelect)+np,indListSelect,optionStruct); %distance based marching
[~,~,ind2]=unique(seedIndex);

Visualization

cFigure;
subplot(1,3,1); hold on;
title('Seed indices','fontSize',fontSize);
hp(1)=gpatch(F,V,d,'none',1); hp(1).FaceColor='Interp';
hp(2)=plotV(V(indSeeds,:),'k.','MarkerSize',markerSize);
legend(hp,{'Mesh distances','Seed point(s)'},'Location','SouthOutSide');
axisGeom;
camlight headlight;
colormap(gca,cMapDist); colorbar;

subplot(1,3,2); hold on;
title('Distances on a triangulated surface model','fontSize',fontSize);
hp(1)=gpatch(F,V,ind2,'none',1);
hp(2)=plotV(V(indSeeds,:),'k.','MarkerSize',markerSize);
legend(hp,{'Mesh seed indices','Seed point(s)'},'Location','SouthOutSide');
axisGeom;
camlight headlight;
colormap(gca,cMapIndices);

subplot(1,3,3); hold on;
title('Resampled surface model','fontSize',fontSize);
plotV(V(indSeeds,:),'k.','MarkerSize',50);
hp(1)=gpatch(F,V,'kw','none',0.5);
hp(2)=gpatch(Fn,Vn,'gw','k',1,2);
legend(hp,{'Original mesh','Resampled mesh','Seed point(s)'},'Location','SouthOutSide');

axisGeom;
camlight headlight;
drawnow;

Warning: Ignoring extra legend entries. 

GIBBON www.gibboncode.org

Kevin Mattheus Moerman, [email protected]

GIBBON footer text

License: https://github.com/gibbonCode/GIBBON/blob/master/LICENSE

GIBBON: The Geometry and Image-based Bioengineering add-On. A toolbox for image segmentation, image-based modeling, meshing, and finite element analysis.

Copyright (C) 2019 Kevin Mattheus Moerman

This program is free software: you can redistribute it and/or modify it under the terms of the GNU General Public License as published by the Free Software Foundation, either version 3 of the License, or (at your option) any later version.

This program is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE. See the GNU General Public License for more details.

You should have received a copy of the GNU General Public License along with this program. If not, see http://www.gnu.org/licenses/.

Published with MATLAB® R2019a